Department of petroleum resources (DPR) Physics Past questions & Answers PDF and Image Download Equations
This is the original complete past question on Department of petroleum resources (DPR) Physics you have been searching for to enable you get a job at DPR Recruitment. Furthermore, here are Searches related to DPR Past Question and Answer; department of petroleum resources past questions, dpr aptitude test pdf dpr test questions nairaland, dpr past questions nairaland, dpr aptitude test format, dpr aptitude test questions, department of petroleum resources past questions pdf and dpr past questions pdf.
As usual. Martins Library is interested in seeing that Nigerian Youths are gainfully engaged in meaningful jobs basically through entrepreneurship or other ways. We feature articles that are directed towards achieving this goal.
However, this article features links which help prepare our subscribers for the FIRS aptitude test, DPR exam and other test that they may be preparing for. Above all, Follow the links to get all the necessary materials of your interest.
However, this article features links which help prepare our subscribers for the FIRS aptitude test, DPR exam and other test that they may be preparing for. Above all, Follow the links to get all the necessary materials of your interest.
Kirchhoff’s Rules
- The sum of the currents entering a junctions is equal to the sum of the currents leaving the junction.
- The sum of the potential differences across all the elements around a closed loop must be zero.
Evaluating Circuits Using Kirchhoff’s Rules
- Assign current variables and direction of flow to all branches of the circuit. If your choice of direction is incorrect, the result will be a negative number. Derive equation(s) for these currents based on the rule that currents entering a junction equal currents exiting the junction.
- Apply Kirchhoff’s loop rule in creating equations for different current paths in the circuit. For a current path beginning and ending at the same point, the sum of voltage drops/gains is zero. When evaluating a loop in the direction of current flow, resistances will cause drops (negatives); voltage sources will cause rises (positives) provided they are crossed negative to positive—otherwise they will be drops as well.
- The number of equations should equal the number of variables. Solve the equations simultaneously.








Proton = 1.602 19 × 10-19 C = 1.67 × 10-27 kg
Neutron = 0 C = 1.67 × 10-27 kg
6.022 × 1023 atoms in one atomic mass unit e is the elementary charge: 1.602 19 × 10-19 C
Potential Energy, velocity of electron: PE = eV = ½mv2
1V = 1J/C 1N/C = 1V/m 1J = 1 N·m = 1 C·V
1 amp = 6.21 × 1018 electrons/second = 1 Coulomb/second
1 hp = 0.756 kW 1 N = 1 T·A·m 1 Pa = 1 N/m2
Power = Joules/second = I2R = IV [watts W]
Quadratic Equation: x b b ac a = - ± 2 - 4 2
Kinetic Energy [J]
KE mv = 12
Interview Questions Tests & Performance (Assessment Interview Preparation)
RWE NPower Test Questions & Answers 2017 | Online Recruitment Aptitude Preparation
2 [Natural Log: when eb = x, ln x = b ]
m: 10-3 m: 10-6 n: 10-9 p: 10-12 f: 10-15 a: 10-18
Addition of Multiple Vectors: r r r r
Rr = Ar+ B +r C r Resultant = Sum of the vectors
R A B C x x x x = + + x-component A A x = cos q r r r r
R A B C y y y y = + + y-component A A y = sin q
R Rx Ry = 2 + 2 Magnitude (length) of R
qR y x R R = tan-1 or tanqR
y x R R = Angle of the resultant
Multiplication of Vectors:
Cross Product or Vector Product:
i ´ j = k j ´ i = -k
i ´ i = 0
Positive direction: i j k
Dot Product or Scalar Product:
i × j = 0 i × i = 1
a × b = abcosq k i j
Derivative of Vectors:
Velocity is the derivative of position with respect to time:
v = + + k = i + j + k
Acceleration is the derivative of velocity with respect to
time: a = + + k = i + j + k
x y z dt
x y z ( i j )
Rectangular Notation: Z = R ± jX where +j represents inductive reactance and -j represents capacitive reactance. For example, Z = 8 + j6W means that a resistor of 8W is in series with an inductive reactance of 6W.
Polar Notation: Z = M Ðq, where M is the magnitude of the reactance and q is the direction with respect to the horizontal (pure resistance) axis. For example, a resistor of 4W in series with a capacitor with a reactance of 3W would be expressed as 5 Ð-36.9° W.
In the descriptions above, impedance is used as an example. Rectangular and Polar Notation can also be used to express amperage, voltage, and power. To convert from rectangular to polar notation:
Given: X - jY (careful with the sign before the ”j”) Magnitude: X 2 + Y2 = M
Angle: tanq = - Y X (negative sign carried over from rectangular notation in this example)
Note: Due to the way the calculator works, if X is negative, you must add 180° after taking the inverse tangent. If the result is greater than 180°, you may optionally subtract 360° to obtain the value closest to the reference angle. To convert from polar to rectangular (j) notation:
Given: M Ðq
X Value: Mcosq
Y (j) Value: Msinq
In conversions, the j value will have the same sign as the q value for angles having a magnitude < 180°. Use rectangular notation when adding and subtracting. Use polar notation for multiplication and division. Multiply in polar notation by multiplying the magnitudes and adding the angles. Divide in polar notation by dividing the magnitudes and subtracting the denominator angle from the numerator angle
MAGNETISM
André-Marie Ampére is credited with the discovery of electromagnetism, the relationship between electric currents and magnetic fields.
Heinrich Hertz was the first to generate and detect electromagnetic waves in the laboratory.
Magnetic Force acting on a charge q: [Newtons N]
F = qvBsinq
F = qv ´ B
F = force [N]
q = charge [C]
v = velocity [m/s]
B = magnetic field [T]
q = angle between v and B
Right-Hand Rule: Fingers represent the direction of the magnetic force B, thumb represents the direction of v (at any angle to B), and the force F on a positive charge emanates from the palm. The direction of a magnetic field is from north to south. Use the left hand for a negative charge.
Also, if a wire is grasped in the right hand with the thumb in the direction of current flow, the fingers will curl in the direction of the magnetic field.
In a solenoid with current flowing in the direction of curled fingers, the magnetic field is in the direction of the thumb. When applied to electrical flow caused by a changing magnetic field, things get more complicated. Consider the north pole of a magnet moving toward a loop of wire
(magnetic field increasing). The thumb represents the north pole of the magnet, the fingers suggest current flow in the loop. However, electrical activity will serve to balance the change in the magnetic field, so that current will actually flow in the opposite direction. If the magnet was being withdrawn, then the suggested current flow would be decreasing so that the actual current flow would be in the
direction of the fingers in this case to oppose the decrease.
Now consider a cylindrical area of magnetic field going into a page. With the thumb pointing into the page, this would suggest an electric field orbiting in a clockwise direction. If the magnetic field was increasing, the actual electric field would be CCW in opposition to the increase. An electron in
the field would travel opposite the field direction (CW) and would experience a negative change in potential.
Force on a Wire in a Magnetic Field: [Newtons N]
F = BI lsinq
F = I l ´ B
F = force [N]
B = magnetic field [T]
I = amperage [A]
l = length [m]
q = angle between B and the direction of the current
Torque on a Rectangular Loop: [Newton·meters N·m]
t = NBIAsinq N = number of turns
B = magnetic field [T]
I = amperage [A]
A = area [m2]
q = angle between B and the plane of the loop
Series RCL Circuits:
The Resultant Phasor X X X L C = - is in the direction of the larger reactance and determines whether the circuit is inductive or capacitive. If XL is larger than XC, then the circuit is inductive and X is a vector in the upward direction.
In series circuits, the amperage is the reference (horizontal) vector. This is observed on the oscilloscope by looking at the voltage across the resistor. The two vector diagrams at right illustrate the phase relationship between voltage, resistance, reactance, and amperage.
XC XL I R VL VC I VR
Series RCL
Impedance Z R X X L C
2 = 2 + ( - )2 Z
R = cosf
Impedance may be found by adding the components using vector algebra. By converting the result to polar notation, the phase angle is also found. For multielement circuits, total each resistance and reactance before using the above formula.
To find total current and phase angle in multielement circuits, find I for each path and add vectorally. Note that when converting between current and resistance, a division will take place requiring the use of polar notation and resulting in a change of sign for the angle since it will be divided into (subtracted from) an angle of zero.
Equivalent Series Circuit: Given the Z in polar notation of a parallel circuit, the resistance and reactance of the equivalent series circuit is as follows: R ZT = cosq X ZT = sinq
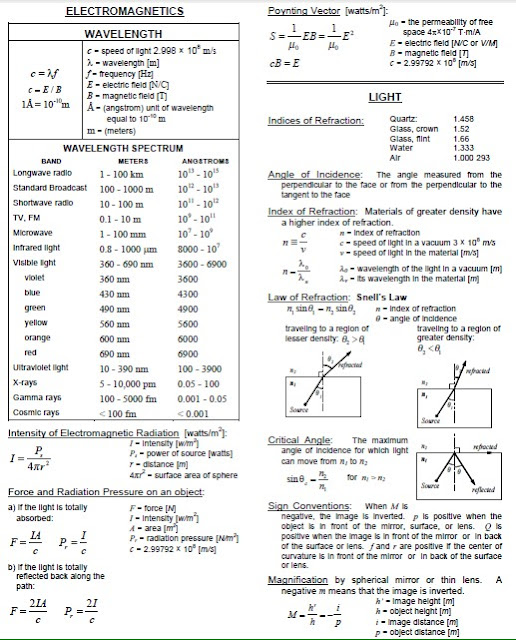
Ggenerally speaking, a reflected ray undergoes a phase shift of 180° when the reflecting material has a greater index of refraction n than the ambient medium. Relative to the same ray without phase shift, this constitutes a path difference of l/2. Interference between Reflected and Refracted rays from a thin material surrounded by another medium:
Constructive: 2 12 nt = (m+ )l
Destructive: 2nt = ml
n = index of refraction
t = thickness of the material [m]
m = fringe order number [integer]
l = wavelength of the light [m]
In conclusion, if the thin material is between two different media, one with a higher n and the other lower, then the above constructive and destructive formulas are reversed. Wavelength within a medium:
l = wavelength in free space [m]
ln = wavelength in the medium [m]
n = index of refraction
c = the speed of light 3.00 × 108 [m/s]
f = frequency [Hz]
Finally, Polarizing Angle: by Brewster’s Law, the angle of incidence that produces complete polarization in the reflected light from an amorphous material such as glass.
